Drag Coefficient
Drag Coefficient
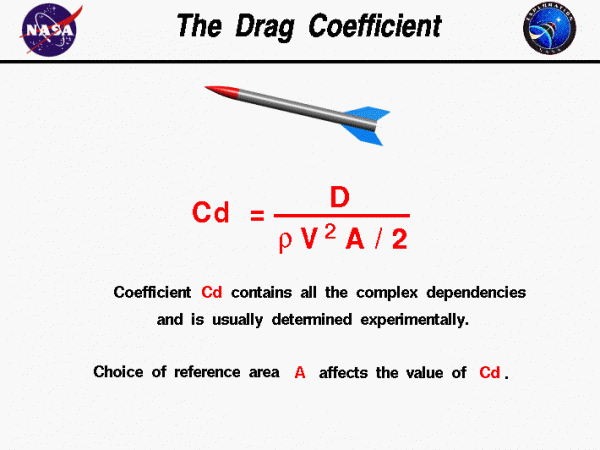
The drag coefficient is a number that engineers use to model all of the complex dependencies of shape and flow conditions on rocket drag. This equation is simply a rearrangement of the drag equation where we solve for the drag coefficient in terms of the other variables. The drag coefficient Cd is equal to the drag D divided by the quantity: density \(\bf \rho\) times half the velocity V squared times the reference area A.
\(\LARGE \mathit{Cd}=\frac{D}{\frac{1}{2}A\rho V^{2}}\)
The quantity one half the density times the velocity squared is called the dynamic pressure q. So
\(\LARGE \mathit{Cd}=\frac{D}{qA}\)
The drag coefficient then expresses the ratio of the drag force to the force produced by the dynamic pressure times the area.
Determining Value for Drag Coefficient
This equation gives us a way to determine a value for the drag coefficient. In a controlled environment like a wind tunnel we can set the velocity, density, and area and measure the drag produced. Through division we arrive at a value for the drag coefficient. As pointed out on the drag equation slide, the choice of reference area (frontal area or surface area) will affect the numerical value of the drag coefficient that is calculated. When reporting drag coefficient values, it is important to specify the reference area that is used to determine the coefficient. We can predict the drag that will be produced under a different set of velocity, density (altitude), and area conditions using the drag equation.
The drag coefficient contains not only the complex dependencies of object shape, but also the effects of air viscosity and compressibility. To correctly use the drag coefficient, we must be sure that the viscosity and compressibility effects are the same between our measured case and the predicted case. Otherwise, the prediction will be inaccurate. For very low speeds (< 200 mph) the compressibility effects are negligible. At higher speeds, it becomes important to match Mach numbers between the two cases. Mach number is the ratio of the velocity to the speed of sound. At supersonic speeds, shock waves will be present in the flow field and we must be sure to account for the wave drag in the drag coefficient. So it is completely incorrect to measure a drag coefficient at some low speed (say 200 mph) and apply that drag coefficient at twice the speed of sound (approximately 1,400 mph, Mach = 2.0). It is even more important to match air viscosity effects. The important matching parameter for viscosity is the Reynolds number that expresses the ratio of inertial forces to viscous forces. In our discussions on the sources of drag, recall that skin friction drag depends directly on the viscous interaction of the object and the flow. If the Reynolds number of the experiment and flight are close, then we properly model the effects of the viscous forces relative to the inertial forces. If they are very different, we do not correctly model the physics of the real problem and will predict an incorrect drag.
