Lift to Drag Ratio
Four Forces
There are four forces that act on an aircraft in flight: lift, weight, thrust, and drag. Forces are vector quantities having both a magnitude and a direction. The motion of the aircraft through the air depends on the relative magnitude and direction of the various forces. The weight of an airplane is determined by the size and materials used in the airplane’s construction and on the payload and fuel that the airplane carries. The weight is always directed towards the center of the earth. The thrust is determined by the size and type of propulsion system used on the airplane and on the throttle setting selected by the pilot. Thrust is normally directed forward along the center-line of the aircraft. Lift and drag are aerodynamic forces that depend on the shape and size of the aircraft, air conditions, and the flight velocity. Lift is directed perpendicular to the flight path and drag is directed along the flight path.
L/D Ratio
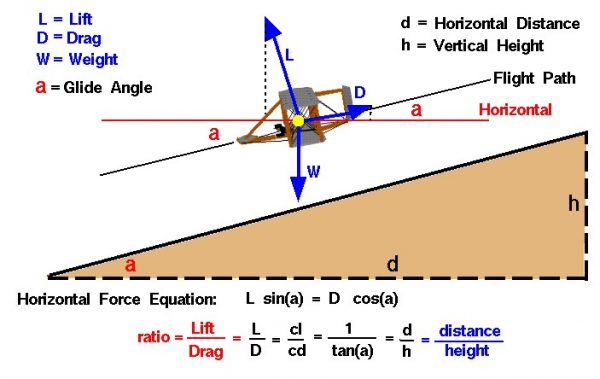
Because lift and drag are both aerodynamic forces, the ratio of lift to drag is an indication of the aerodynamic efficiency of the airplane. Aerodynamicists call the lift to drag ratio the L/D ratio, pronounced “L over D ratio.” An airplane has a high L/D ratio if it produces a large amount of lift or a small amount of drag. Under cruise conditions lift is equal to weight. A high lift aircraft can carry a large payload. Under cruise conditions thrust is equal to drag. A low drag aircraft requires low thrust. Thrust is produced by burning a fuel and a low thrust aircraft requires small amounts of fuel be burned. As discussed on the maximum flight time page, low fuel usage allows an aircraft to stay aloft for a long time, and that means the aircraft can fly long range missions. So an aircraft with a high L/D ratio can carry a large payload, for a long time, over a long distance. For glider aircraft with no engines, a high L/D ratio again produces a long range aircraft by reducing the steady state glide angle at which the glider descends.
Lift Equation
As shown in the middle of the slide, the L/D ratio is also equal to the ratio of the lift and drag coefficients. The lift equation indicates that the lift L is equal to one half the air density rho (ρ) times the square of the velocity V times the wing area A times the lift coefficient Cl:
\(\LARGE L=C_l\cdot\frac{\rho\cdot V^2\cdot A}2\)
Drag Equation
Similarly, the drag equation relates the aircraft drag D to a drag coefficient Cd:
\(\LARGE D=C_d\cdot\frac{\rho\cdot V^2\cdot A}2\)
Dividing these two equations give:
\(\LARGE \frac LD=\frac{C_l}{C_d}\)
Lift and drag coefficients are normally determined experimentally using a wind tunnel. But for some simple geometries, they can be determined mathematically.
Application
This figure at the top of this page shows the balance of forces on a descending Wright 1902 glider. The flight path of the glider is along the thin black line, which falls to the left. The flight path intersects the horizontal, thin, red line at an angle “a” called the glide angle.
The tangent of the glide angle, tan(a), is equal to the vertical height (h) which the aircraft descends divided by the horizontal distance (d) which the aircraft flies across the ground.
\(\LARGE \tan(a)=\frac{h}{d}\)
The tangent of the glide angle is also related to the ratio of the drag, D, of the the aircraft to the lift, L.
\(\LARGE \frac{D}{L}=\frac{c_d}{c_l}=\tan(a)\)
Where cd and cl are the drag and lift coefficients derived from the drag and lift equations and measured during wind tunnel testing.
What good is all this for aircraft design? If we combine the two equations into a single equation through the tan(a), and invert the equation, we get:
\(\LARGE \frac{L}{D}=\frac{c_l}{c_d}=\frac{d}{h}=\frac{1}{\tan(a)}\)
The lift divided by drag is called the L/D ratio, pronounced “L over D ratio.” From the last equation we see that the higher the L/D, the lower the glide angle, and the greater the distance that a glider can travel across the ground for a given change in height. Because lift and drag are both aerodynamic forces, we can think of the L/D ratio as an aerodynamic efficiency factor for the aircraft. Designers of gliders and designers of cruising aircraft want a high L/D ratio to maximize the distance which an aircraft can fly. It is not enough to just design an aircraft to produce enough lift to overcome weight. The designer must also keep the L/D ratio high to maximize the range of the aircraft.
We could have used D/L as the efficiency factor, but then the lower the factor the better the aircraft. Engineers usually define efficiency factors so that “bigger” is “better”.